By Andrew R. Melendrez, Department of Physics, Colorado School of Mines
ABSTRACT
This study examined the pressure on the bottom layer of a pallet of a prominent beverage company’s twelve-ounce aluminum cans. The measured pressure is specific to loading and unloading of the product from a forklift using a slip-sheet as the pallet’s floor support and as a handle for transportation. The pressure is measured using Pressurex tactile pressure sensor film (“TPSF”)3, which is a Mylar film containing a layer of tiny color filled microcapsules that rupture when a force is applied. The intensity of these red color ruptures is in direct correlation to the applied force. Observations in the company’s warehouse have reported that the bottom layer of the pallet’s cans have received damage and are the reason for this examination. To reduce this damage most effectively, an increase of the time interval in which the pallet is loaded onto the forklift should be implemented.
INTRODUCTION
The research objectives are to measure the pressure on the bottom layer of a pallet as it is loaded and unloaded from a forklift in order to identify the variables associated with product damage. Pressure was measured using a special encapsulated Mylar film known as Pressurex TPSF. Other methods like stress gauges and pressure meters are somewhat expensive and require training. Pressurex TPSF, on the other hand, works like making a carbon copy. The film comes in two separate sheets that are placed together. One sheet contains a several layers of tiny microcapsules of red dye that rupture when a pressure above 350 psi is applied. The second sheet is a white paper with a Mylar back. The white paper is mated to the first sheet and receives the color from the microcapsules. The intensity of the red color increases as more capsules in each layer rupture. The intensity of the color is therefore in direct correlation to the pressure applied. Low pressure range film (350 psi to 1400 psi) was the recording medium in this experiment. The film was glued the film down the center of the slip-sheet to measure pressure during the entire loading and unloading process. This combination of slip-sheet and film was positioned on the bottom of a pallet; the pallet was loaded and unloaded by a forklift operator. Once completed, the Pressurex film (now developed) was removed and for optical image analysis.
THEORY
The kinematics of the pallet can be calculated using the impulse momentum theorem.1 The theorem states, “the change in momentum of a body during a time interval equals the impulse of the net force that acts on the body during that interval.” We define J as the impulse momentum by:
![]()
Calculation 1
where p2 and p1 are two different momentums. The change in momentum is due to a “ripple” impulse as the columns of the pallet move onto and off of the forklift’s inclined platform (Figure 1). The impulse is due to a change in velocity because the pallet is forced to alter its direction.

Figure 1
Figure 1 shows the pallet initially with a horizontal velocity parallel with the ground vi and then changes direction to movement at an angle Θ relative to the ground along the forklift’s platform. The sudden change in direction is the ripple impulse and can be characterized by vector analysis of the velocities. The components of the pallet’s velocity were defined using Cartesian coordinates, where vx is velocity parallel to the ground and vy is velocity perpendicular to the ground (Figure 1). The change in velocity can be characterized by the change in momentum for each coordinate direction and then used as the impulse momentum in Equation (1). The force F that the forklift applies to the pallet is
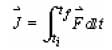
Calculation 2
where the integral over t is the time interval during which the force is applied.1 Equation (2) is used in each coordinate direction to solve for the force. The average force Fave
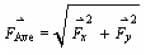
Calculation 3
is the magnitude of the forces. Equations (1) and (2) were combined because they show how the variable time and angle have on the force. These are also the variables that can be altered and tested in the warehouse. The constants in the momentum are the mass of the pallet, m, and the length of the pallet l, where the initial velocity is the distance the pallet moved or length divided by the time.
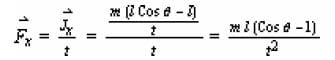
Calculation 4
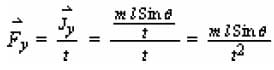
Calculation 5
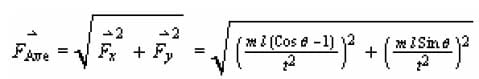
Calculation 6
The Taylor expansion approximation for the cosine of a small angle as cosΘ ≈ 1- Θ2/2 and sin2Θ + cos2Θ = 1 was employed; thus. the average force can be simplified to reduce the radical.1 The resulting equation
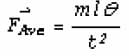
Calculation 7
shows the direct and indirect relationships to the average force. Using Wolfram Mathematica4, a 3D plot of the average force (Figure 2) was made.
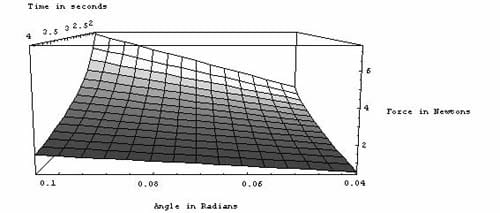
Figure 2
Figure 2 graphically shows that the relationship between the force and the angle is linear. Figure 2 also shows the time is quadradically related to the force by the graph’s sharp rise as time decreases. Therefore, to reduce can deterioration due to high forces, as seen in the warehouse, the time interval for a pallet being loaded onto a forklift should be increased.
PROCEDURE
A test matrix for the Pressurex film to test the theory of the relationship between the time and angle to the average force was set up. I planned my test to measure the forces at the extremes of the two variables.
| High angle of 6° and short time below 2 s. | High angle of 6° and long time abov e 3.5 s. |
| Low angle of 2.3° and short time below 2 s. | Low angle of 2.3° and long time above 3.5 s. |
Using the test matrix four pallets with Pressurex film were instrumented. The film was placed down the center of the slip-sheet between the pallet and the slip-sheet. A forklift operator then loaded the test pallet accordingly. The forklift operator only loaded and unloaded each pallet once and the film was removed to stop recording.
RESULTS
The film was returned to SPI for optical image analysis, which would provide more accurate results than just viewing the intensity of the color on the film with the naked eye. In order for the film to fit in the scanner each pallet test had to be cut into three sections representing the front, middle, and back of the pallet. Each section was analyzed separately and added together with the corresponding sections to form the statistics for the trial. The film measures pressures from a range of 355 psi to 1565 psi and the analysis outputs a histogram. The histogram shows the percentage of the forces applied to the film. The pressures are broken into twenty-nine bins over the range of the film and the percentages of the pressures sums to 100 percent of the total scan. Figure 3 is the histogram of pressure for the front section of the pallet in the high angle and low time trial.
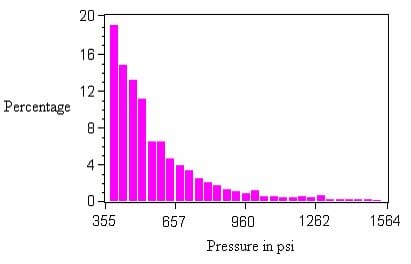
Figure 3. Pressure Histogram
This histogram shows that the majority of the pressure is at the low end of the range. The front, middle, and back sections of each trial had a histogram of pressure similar to Figure 3. The pressures in each of the trials were summed over the sections and the weighted average µ with respect to the percentage for the entire trial was calculated using x as the pressure and w as the percentage.2
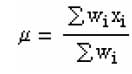
Calculation 8
The weighted variance in the data δ2
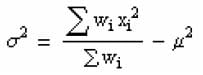
Calculation 9
is calculated using the weighted average.2 The results of each trial were calculated along with the uncertainty in the analysis.
| Weighted average pressure (PSI) | Weighted uncertainty δ | Area of pressure distribution (in) | Average Force (lb) | |
| High angle and short time | 573.41 | 288.1948 | 14.99 | 8767.4 |
| High angle and long time | 550.9 | 217.82 | 7.17 | 3949.9 |
| Low angle and short time | 532.344 | 203.51 | 2.68 | 1426.8 |
| Low angle and long time | 573.98 | 233.49 | 1.52 | 872.4 |
Results of the force measured by the film along with the associated time and angle the pallets were loaded for each test were then plotted using the matrix.
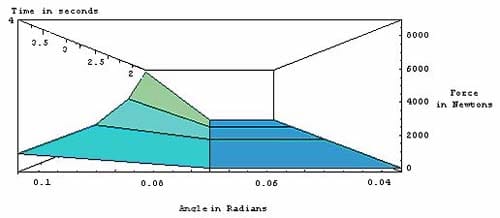
Figure 4
With this data Wolfram Mathematica could then be employed to make a least-squares fit and solve for the variables time and angle. The fit was 4680 Θ/t2 and is plotted in Figure 5.
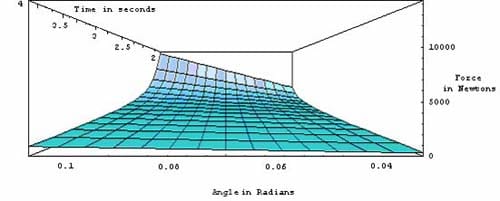
Figure 5
Figure 5 is similar to the graph that was plotted in the theory. They correspond extremely well and prove that the relation ship between the force and angle is linear while the time dependence is quadratic. I plotted an array of both fit and measured graphs on the same plot in two views in Figure 6 and Figure 7.
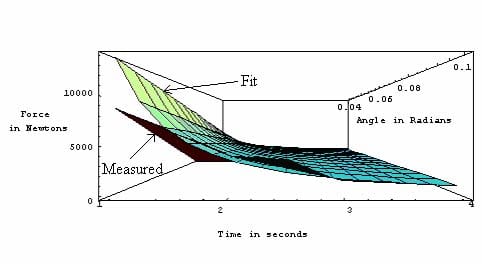
Figure 6
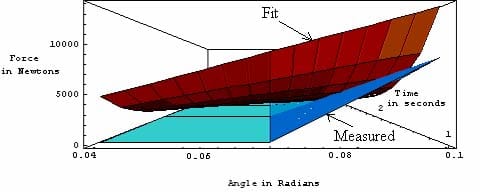
Figure 7
Figure 7 displays that the measured plot and the fit graph have close to the same slope. Therefore the fit agrees very closely to the measured data.
CONCLUSION
The results from the film proved the theory about the relationship between the variables of time and angle to the average force a pallet experiences. The best way to reduce the force will be to increase the time interval in which the pallet is loaded onto the forklift. As of when this paper was written, the average pallet load time is 2.3 s. If this time were increased to 3.5 s the force the pallet experiences would be cut in half. Process standards can now be implemented using the results of this examination to reduce can damage and increase product quality.
REFERENCES
- Young, H. D., and Freedman, R. A. (1996). University Physics 9th Edition. Reading: Addison-Wesley.
- Bevington, P. R., and Robinson, D. K. (1992). Data Reduction and Error Analysis for the Physical Sciences Boston: McGraw-Hill.
- Pressurex, (2002). East Hanover, NJ: Sensor Products Inc.
- Mathematica Version 4 [Computer Program]. (1988). Champaign, IL: Wolfram Research Inc.


